how to find the equation of a parabola

Find Equation of a Parabola from a Graph

Several methods are used to find equations of parabolas given their graphs. Examples are presented along with their detailed solutions and exercises.

Examples with Detailed Solutions

Example 1 Graph of parabola given x and y intercepts
Find the equation of the parabola whose graph is shown below.

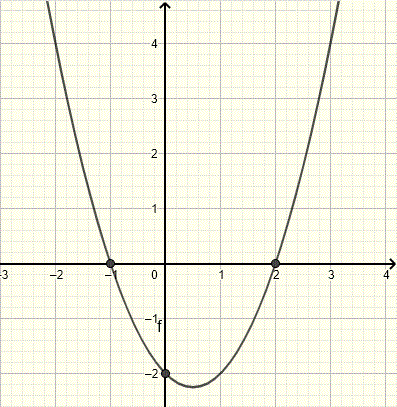
graph of parabola for example 1

Solution to Example 1

The graph has two x intercepts at \( x = - 1 \) and \( x = 2 \). Hence the equation of the parabola may be written as
\( y = a(x + 1)(x - 2) \)
We now need to find the coefficient \( a \) using the y intercept at \( (0,-2) \)
\( -2 = a(0 + 1)(0 - 2) \)
Solve the above equation for \( a \) to obtain
\( a = 1 \)
The equation of the parabola whose graph is given above is
\( y = (x + 1)(x - 2) = x^2 - x - 2\)

Example 2 Graph of parabola given vertex and a point
Find the equation of the parabola whose graph is shown below.

graph of parabola for example 2

Solution to Example 2

The graph has a vertex at \( (2,3) \). Hence the equation of the parabola in vertex form may be written as
\( y = a(x - 2)^2 + 3 \)
We now use the y intercept at \( (0,- 1) \) to find coefficient \( a \).
\( - 1 = a(0 - 2) + 3\)
Solve the above for \( a \) to obtain
\( a = 2 \)
The equation of the parabola whose graph is shown above is
\( y = 2(x - 2)^2 + 3\)

Example 3 Graph of parabola given three points
Find the equation of the parabola whose graph is shown below.

graph of parabola for example 3

Solution to Example 3

The equation of a parabola with vertical axis may be written as
\( y = a x^2 + b x + c \)
Three points on the given graph of the parabola have coordinates \( (-1,3), (0,-2) \) and \( (2,6) \). Use these points to write the system of equations
\( \begin{array}{lcl} a (-1)^2 + b (-1) + c & = & 3 \\ a (0)^2 + b (0) + c & = & -2 \\ a (2)^2 + b (2) + c & = & 6 \end{array} \)
Simplify and rewrite as
\( \begin{array}{lcl} a - b + c & = & 3 \\ c & = & -2 \\ 4 a + 2 b + c & = & 6 \end{array} \)
Solve the above 3 by 3 system of linear equations to obtain the solution
\( a = 3 , b=-2 \) and \(c=-2 \)
The equation of the parabola is given by
\( y = 3 x^2 - 2 x - 2 \)

Example 4 Graph of parabola given diameter and depth
Find the equation of the parabolic reflector with diameter D = 2.3 meters and depth d = 0.35 meters and the coordinates of its focus.

graph of parabolic reflector for example 4

Solution to Example 4

The parabolic reflector has a vertex at the origin \( (0,0) \), hence its equation is given by
\( y = \dfrac{1}{4p} x^2 \)
The diameter and depth given may be interpreted as a point of coordinates \( (D/2 , d) = (1.15 , 0.35) \) on the graph of the parabolic reflector. Hence the equation
\( 0.35 = \dfrac{1}{4p} (1.15)^2 \)
Solve the above equation for \( p \) to find
\( p = 0.94 \)
The equation of the parabola is given by
\( y = 0.26 x^2 \)
The focus of the parabolic reflector is at the point
\( (p , 0) = (0.94 , 0 ) \)